A typical question to accompany a question like this might be 'How many squares are in the hundredth term?' or even 'what is the nth term?' In the past, I have dutifully created a table of values and used all sorts of algebraic techniques to come up with such an algebraic rule. This is fine, to an extent, but if this is all we do (or get our students to do) then we are missing out on a grand opportunity to do some beautiful maths.
So why does Dan get so excited about this pattern? Think about how many different ways it can be seen:
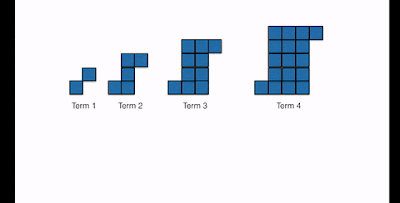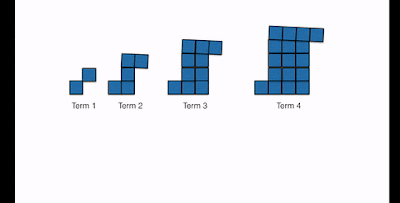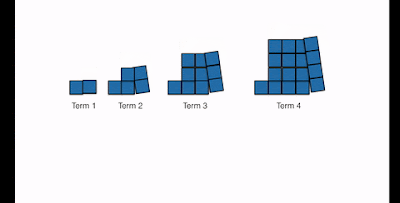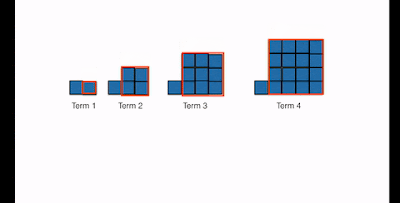
Perhaps you see it as an inner rectangle and two squares. Generalising this suggests an nth term of (n-1)(n+1)+2
Maybe you see an inner square and two identical rectangles. Generalising, this suggests an nth term of (n-1)2+2n
Maybe you see an opportunity to complete an 'outer' square with two identical rectangles. Generalising, this suggests an nth term of (n+1)2– 2n
Or maybe you transformed the pattern by taking the top layer of each term, rotating it 90˚ to create a large square and a small square.
Generalising, this suggests an nth term of n2+1.
In fact, much fun can be had showing that all these general terms do simplify to n2+1.
Using decomposing strategies like those shown above, how can we describe the hundredth term? the nth term? How many ways can we see this?
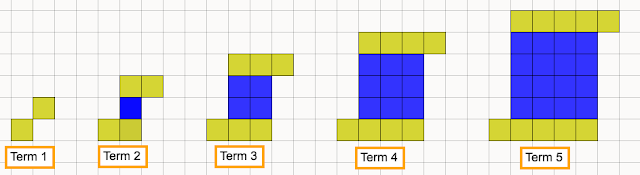
I showed it to Dan. He liked it (how much I'm not sure) and funnily enough, he saw it in a different way than I. He then set about creating a 'decomposable' visual pattern of his own:
So, be honest, which of these is the most joyous?
* * *
These diagrams were created using the Colour Tiles from the mathies.ca site.







No comments:
Post a Comment