I ♥ Arrays

Arrays are brilliant.
I'll say that again.
Arrays are brilliant. I love the fact that I can talk about them in a kindergarten class as well as in a Grade 12 class. Arrays allow me as a teacher to help students connect ideas and concepts especially when it comes to multiplicative thinking. Yet I had no real experience of arrays at school but I wish I did; it would have given me a much deeper understanding of some important algebraic concepts.
 
So I was in a Grade 5 class this week. The teacher has been working very hard to address any gaps in the students' additive thinking and was now pretty sure that they were ready for some work on multiplication We wanted to see how they would solve a fairly routine equal groups problem so gave them the following:

A baking tray holds 15 donuts. How many donuts would there be on 13 trays?
 
Now one or two attempted to use an algorithm but were making lots of procedural errors:

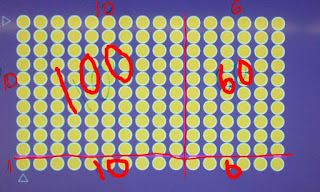
Others relied on sketching out the problem:

In the case above the thirteen trays drawn have 10 donuts giving 130. The student then reasoned that the remaining five donuts per tray would give another 65 thus giving 195 in total.
Others used some really neat student-generated procedures:

What was important was that the students sensed that they had to do 15×13. They had also done some work using arrays to represent 6×5, 4×3 etc. So I said that suppose we had to do 16×11 then we would get an array like:

 

By splitting the 16 and 11 into a friendlier 10 and 6, and 10 and 1 the students were able to tell me quickly how much was in each of the four quadrants:

... and from there tell me that the answer was 176 (some added in their head, others wrote their work on paper). We then returned to the donuts question to see how we could use the array to help us here but I pointed out that I didn't want to draw out all the donuts as it would be too time-consuming!

"Wow, that's so much quicker," said one student and many agreed. We consolidated by trying another problem (essentially 17×15) and here is a sample of work:



I love the way that there are no place value lies in this method and that it encourages good number sense. I have seen some students develop this array method into a partial products method:



...which is neat but to me what is even neater is when I can use the array method to explain what happens when you multiply polynomials. When I first came to Canada I learned a new acronym: FOIL. I found a lot of students misunderstood this (it stands for First, Outside, Inside, Last) but they had much greater success when they drew an array:

And when there are more terms in the polynomials it becomes easier to collect the like terms as they lie on the diagonals:

I have shared this method with parents at Math Evenings and it is always neat to see their faces when I give these examples: they finally understand why it works!
I could go on about how arrays make it easier to understand what it means to 'complete the square' or how they can be used to do polynomial division (see James Tanton's excellent video on this).
But for today, it was rewarding to see how quickly the students took to this method and how it fitted in so nicely with their existing knowledge.